Solve the problem.What conditions, when present, are sufficient to conclude that a function f(x) has a limit as x approaches some value of a?
A. f(a) exists, the limit of 
as 
from the left exists, and the limit of 
as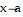 
from the right exists.
B. The limit of 
as 
from the left exists, the limit of 
as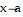 
from the right exists, and at least one of these limits is the same as f(a).
C. The limit of 
as 
from the left exists, the limit of 
as 
from the right exists, and these two limits are the same.
D. Either the limit of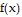 
as 
from the left exists or the limit of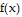 
as 
from the right exists
Answer: C
You might also like to view...
Provide an appropriate response.Obtain the first nonzero term of the Maclaurin series for sin(tan x) - tan(sin x).
A.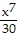 
B.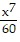 
C. -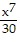 
D. -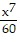 
Perform the division.
A. y2 + 2
B. y - 2
C. y + 
D. y + 2
Solve the problem.Find the exact value of x in the figure.
A. 11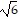
B.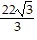 
C. 
D. 11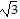
Translate the percent problem to a basic percent equation using x for the unknown value. Do not solve.76 out of what number is 38%?
A. x ? 38 = 76 B. 0.38 ÷ x = 76 C. 76 ? 0.38 = x D. x ? 0.38 = 76