Solve the problem.A hiker is at point A as shown in the graph and wants to take the shortest route through a field to reach a nearby road represented by  . The shortest route will be to walk perpendicular to the road. Is
. The shortest route will be to walk perpendicular to the road. Is 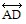 the shortest route? Explain.
the shortest route? Explain.
A. The product of the slopes of the two lines is 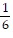 ?
?  , which is equal to -1, so
, which is equal to -1, so  is perpendicular to
is perpendicular to 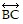 and is therefore the shortest route.
and is therefore the shortest route.
B. The product of the slopes of the two lines is 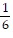 ?
?  , which is equal to -1, so
, which is equal to -1, so 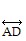 is not perpendicular to
is not perpendicular to 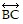 and is therefore not the shortest route.
and is therefore not the shortest route.
C. The product of the slopes of the two lines is 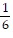 ? 6, which is equal to 1, so
? 6, which is equal to 1, so  is not perpendicular to
is not perpendicular to 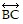 and is therefore not the shortest route.
and is therefore not the shortest route.
D. The product of the slopes of the two lines is  ? 6, which is equal to 1, so
? 6, which is equal to 1, so 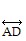 is perpendicular to
is perpendicular to 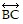 and is therefore the shortest route.
and is therefore the shortest route.
Answer: A
You might also like to view...
Use the total differential dz to approximate the change in z as (x, y) moves from P to Q. Then use a calculator to find the corresponding exact change ?z (to the accuracy of your calculator).4x3y2; P(1, 1), Q(1.01, 0.99)
A. dz = 0.04; ?z = 0.0381920404 B. dz = 0.04; ?z = 0.0391920404 C. dz = 0.03; ?z = 0.0391920404 D. dz = 0.04; ?z = 0.0416920404
Factor the perfect square trinomial completely.x4 + 10x2 + 25
A. (x2 - 5)2 B. (x2 + 5)2 C. (x2 + 10)(x2 - 10) D. (x2 + 5)(x2 - 5)
Simplify by writing the expression with positive exponents. Assume that all variables represent nonzero real numbers.
A. 
B. 
C. 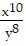
D. 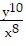
Subtract.(16a4 - 7a2) - (10a4 + 5a2)
A. 26a4 - 2a2 B. 6a4 - 2a2 C. 6a4 - 12a2 D. -6a6