Give the exact value.tan 60°
A. 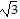
B. 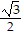
C. 2
D. 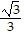
Answer: A
You might also like to view...
Solve the differential equation.ex + 4ex y = 3, x > 0
+ 4ex y = 3, x > 0
A. y = e-4x + Ce-x, x > 0 B. y = ex + Ce-4x, x > 0 C. y = e-x + Ce-4x, x > 0 D. y = e-x + e-4x, x > 0
Provide an appropriate response.Is f continuous at x = 4? 
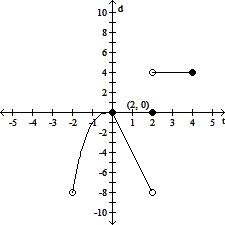
A. Yes B. No
Evaluate the expression.71
A. 7
B. 1
C. -7
D. 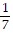
Provide an appropriate response.The parallel dot plots show the number of fatalities per year caused by tornadoes in a certain state for two periods: 1950-1974 and 1975-1999. In addition to comparing these distributions, suggest a reason to explain any differences. 
A. In both periods, the number of fatalities per year ranges from 0 to 5. During the period 1950-1974, the most common number of fatalities was 2. The distribution for this period is roughly symmetric - the number of years having more than 2 fatalities is roughly the same as the numbers of years having fewer than 2 fatalities. During the period 1975-1999, the most common number of fatalities is 0 and most years have 0 or 1 fatality. Only a few years have more than 1 fatality during this period. This could be due to higher construction standards, better warning systems, or medical advancements. B. In both periods, the number of fatalities per year ranges from 0 to 5. During the period 1950-1974, the most common number of fatalities was 2. The distribution for this period is roughly symmetric - the number of years having more than 2 fatalities is roughly the same as the numbers of years having fewer than 2 fatalities. During the period 1975-1999, the most common number of fatalities is 1 and most years have 1 or 2 fatalities. Only a few years have more than 2 fatalities during this period. This could be due to higher construction standards, better warning systems, or medical advancements. C. During the period 1950-1974, the number of fatalities per year ranges from 1 to 5 with the most common number of fatalities being 2. The distribution for this period is roughly symmetric - the number of years having more than 2 fatalities is roughly the same as the numbers of years having fewer than 2 fatalities. During the period 1975-1999, the number of fatalities per year ranges from 0 to 5 with the most common number of fatalities being 0. During this period, most years have 0 or 1 fatality and only a few years have more than 1 fatality. This could be due to higher construction standards, better warning systems, or medical advancements. D. In both periods, the number of fatalities per year ranges from 0 to 5. During the period 1950-1974, the most common number of fatalities was 3. The distribution for this period is roughly symmetric - the number of years having more than 3 fatalities is roughly the same as the numbers of years having fewer than 3 fatalities. During the period 1975-1999, the most common number of fatalities is 0 and most years have 0 or 1 fatality. Only a few years have more than 1 fatality during this period. This could be due to higher construction standards, better warning systems, or medical advancements.