Use the Principle of Mathematical Induction to show that the statement is true for all natural numbers n.1 ? 6 + 2 ? 6 + 3 ? 6 + . . . + 6n = 
What will be an ideal response?
First we show that the statement is true when n = 1.
For n = 1, we get 6 = 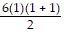 = 6.
= 6.
This is a true statement and Condition I is satisfied.
Next, we assume the statement holds for some k. That is,
 is true for some positive integer k.
is true for some positive integer k.
We need to show that the statement holds for k + 1. That is, we need to show that

So we assume that 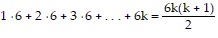 is true and add the next term,
is true and add the next term, to both sides of the equation.
1 ? 6 + 2 ? 6 + 3 ? 6 + . . . + 6k + 6(k + 1) = 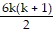 + 6(k + 1)
+ 6(k + 1)
= 
= 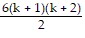
Condition II is satisfied. As a result, the statement is true for all natural numbers n.
You might also like to view...
Complete.9 mi =  yd
yd
A. 47,520
B. 
C. 15,840
D. 9000
Find an equation in slope-intercept form of the line that passes through the given point and has slope m.(9, 4); m is undefined
A. y = 9 B. x = 9 C. x = 4 D. y = 4
Solve the problem.If the area of a rectangle is fixed, the length of the rectangle varies inversely as the width. If the length of a rectangle is 25 inches and its width is 50 inches, find the length of a rectangle with a width of 10 inches.
A. 5 inches
B. 125 inches
C. 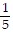 inches
inches
D. 20 inches
The two triangles below are similar. Find the missing lengths.
A. x = 24 B. x = 23 C. x = 30 D. x = 16