Solve the problem.Financial analysts in a company that manufactures ovens arrived at the following daily cost equation for manufacturing x ovens per day: C(x) = x2 + 4x + 1800. The average cost per unit at a production level of x ovens per day is 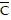 (x) = C(x)/x. (i) Find the rational function
(x) = C(x)/x. (i) Find the rational function  . (ii) Sketch a graph of
. (ii) Sketch a graph of 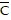 (x) for 10 ? x ? 125. (iii) For what daily production level (to the nearest integer) is the average cost per unit at a minimum, and what is the minimum
(x) for 10 ? x ? 125. (iii) For what daily production level (to the nearest integer) is the average cost per unit at a minimum, and what is the minimum
average cost per oven (to the nearest cent)? HINT: Refer to the sketch in part (ii) and evaluate  (x) at appropriate integer values until a minimum value is found.
(x) at appropriate integer values until a minimum value is found. 
A. (i)  (x) =
(x) = 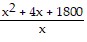
(ii) 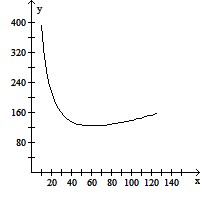
(iii) 61 units; $133.29 per oven
B. (i)  (x) =
(x) = 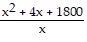
(ii) 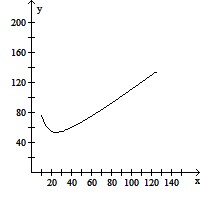
(iii) 22 units; $48.93 per oven
C. (i)  (x) =
(x) = 
(ii) 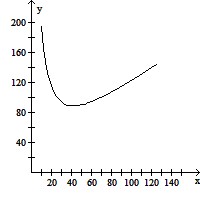
(iii) 42 units; $88.86 per oven
D. (i)  (x) =
(x) = 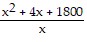
(ii) 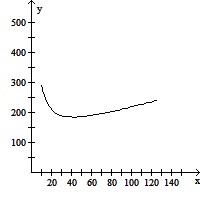
(iii) 44 units; $185.61 per oven
Answer: C
You might also like to view...
Find the vector projv u.v = k, u = 2i + 6j + 9k
A. 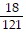 i +
i + 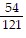 j +
j + 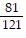 k
k
B. 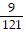 k
k
C. 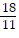 i +
i + 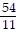 j +
j + 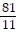 k
k
D. 9k
Provide an appropriate response.
A conduit ABCD must be made so that ?CBE is 45°. If BE = 5 cm and AK = 16 cm, find the length of the conduit to the nearest thousandth of a centimeter. 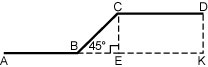
A. 18.071 cm B. 23.071 cm C. 14.536 cm D. 19.660 cm
Solve each problem.If the price charged for a bolt is p cents, then x thousand bolts will be sold in a certain hardware store, where 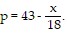 How many bolts must be sold to maximize revenue?
How many bolts must be sold to maximize revenue?
A. 774 bolts B. 387 bolts C. 774 thousand bolts D. 387 thousand bolts
Solve the equation. =
= 
A. 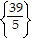
B. 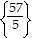
C. 
D. 