Find the vertex form for the quadratic function. Then find each of the following:(A) Intercepts(B) Vertex(C) Maximum or minimum(D) Rangen(x) = -x2 + 8x - 7
A. Standard form: n(x) = -(x + 4)2 + 9
(A) x-intercepts: -7, - 1; y-intercept: -7
(B) Vertex (4, 9)
(C) Maximum: 9
(D) y ? 9
B. Standard form: n(x) = -(x - 4)2 + 9
(A) x-intercepts: 1, 7; y-intercept: -7
(B) Vertex (4, 9)
(C) Maximum: 9
(D) y ? 9
C. Standard form: n(x) = -(x - 4)2 + 9
(A) x-intercepts: 1, 7; y-intercept: -7
(B) Vertex (-4, -9)
(C) Maximum: 9
(D) y ? 9
D. Standard form: n(x) = -(x + 4)2 + 9
(A) x-intercepts: 1, 7; y-intercept: -7
(B) Vertex (4, 9)
(C) Minimum: 9
(D) y ? 9
Answer: B
You might also like to view...
Find the exact value of x.tan-1 x = sin-1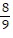 
A.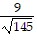 
B.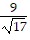 
C. 
D.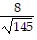 
Solve the problem.The quotient of a number and 2.6 is -16.6. Find the number.
A. -14 B. -43.16 C. -19.2 D. -19.3
Evaluate.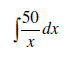 
What will be an ideal response?
A triangle with vertices at (x1, y1), (x2, y2), and (x3, y3) has area equal to the absolute value of D, where D = 
.Find the area of the triangle having vertices at P, Q, and R.P(1, -2), Q(1, 3), R(5, -1)
A. 7 B. 0 C. 20 D. 10