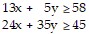Determine whether the two given lines are parallel. 3x - 4y = 1 8x + 6y = 1
A. Yes
B. No
Answer: B
You might also like to view...
Sketch a line passing through the point and having slope m.(0, 5), m = -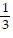 
A.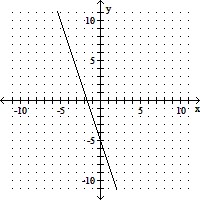 
B. 
C. 
D.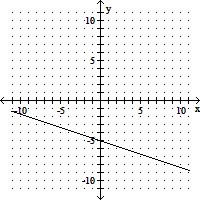 
Use the quadratic formula to solve the quadratic equation.m2 - 12 = m
A. m = -3, -4 B. m = 3, 4 C. m = 1, 12 D. m = -3, 4
Solve the problem.Two foods are to be mixed. Food I contains 10 grams of carbohydrates per unit. Food II contains 5 grams of carbohydrates per unit. The total grams of carbohydrates in the mixture may not exceed 170 grams. No more than 17 units of Food I and no more than 12 units of Food II are to be used in the mixture. Each food contains 20 grams of protein per unit. How many units of each food should be used to maximize the grams of protein in the mixture?
A. 11 units of Food I and 12 units of Food II B. 34 units of Food II and 0 units of Food I C. 13 units of Food I and 8 units of Food II D. 12 units of Food I and 10 units of Food II
Set up the linear programming problem.A dietitian needs to purchase food for patients. She can purchase an ounce of chicken for $0.25 and an ounce of potatoes for $0.02. The dietician is bound by the following constraints.? Each ounce of chicken contains 13 grams of protein and 24 grams of carbohydrates.? Each ounce of potatoes contains 5 grams of protein and 35 grams of carbohydrates.? The minimum daily requirements for the patients under the dietitian's care are 45 grams of protein and 58 grams of carbohydrates. Let x = the number of ounces of chicken and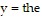 
number of ounces of potatoes purchased per patient. Write a system of inequalities that describes these
constraints.
A. 
B. 
C. 
D. 
