Analyze the graph of the given function f as follows:(a) Determine the end behavior: find the power function that the graph of f resembles for large values of |x|.(b) Find the x- and y-intercepts of the graph.(c) Determine whether the graph crosses or touches the x-axis at each x-intercept.(d) Graph f using a graphing utility.(e) Use the graph to determine the local maxima and local minima, if any exist. Round turning points to two decimal places.(f) Use the information obtained in (a) - (e) to draw a complete graph of f by hand. Label all intercepts and turning points.(g) Find the domain of f. Use the graph to find the range of f.(h) Use the graph to determine where f is increasing and where f is decreasing.f(x) = x2(x + 2)
What will be an ideal response?
(a) For large values of |x|, the graph of f(x) will resemble the graph of y = x3.
(b) y-intercept: (0, 0), x-intercepts: (0, 0) and (-2, 0)
(c) The graph of f crosses the x-axis at (-2, 0) and touches the x-axis at (0, 0).
(e) Local minimum at (0, 0), Local maximum at (-1.33, 1.19)
(f) 
(g) Domain of f: all real numbers; range of f: all real numbers
(h) f is increasing on (-?, -1.33) and (0, ?); f is decreasing on (-1.33, 0)
You might also like to view...
Divide and simplify. Assume that all variables represent positive values.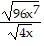
A. 4x3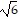
B. 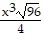
C. 2x3
D. 2x6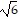
Solve using the quadratic formula. Use a calculator to approximate the solution to three decimal places.2x - 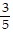 =
=  x2
x2
A. -3.100, -0.233 B. 3 C. -0.333 D. 0.333, 3
Graph.f(x) = 4x2 - 2x - 1
A. 
B. 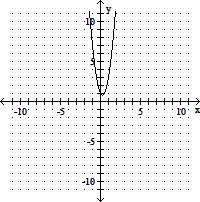
C. 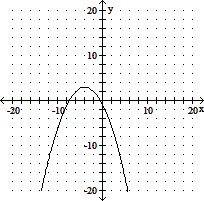
D. 
Solve the equation.-0.5(30,000) + 0.15p = 0.02(30,000 +p)
A. {20,280} B. {1,200,000} C. {2028} D. {120,000}