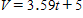If a rock is thrown downward near the surface of Mercury, then the downward velocity V, in meters per second, of the rock after t seconds satisfies the equation of change
.A: What can you conclude about the function 
?
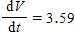
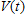
B: If the rock is thrown downward with an initial velocity of 5 meters per second, find a formula that gives the velocity of the rock after t seconds.C: What is the acceleration due to gravity on the surface of Mercury?
What will be an ideal response?
?
B: 
?
C: 3.59 meters per second per second
You might also like to view...
Find the magnitude of u × v and the unit vector parallel to u × v in the direction of u × v.u = -4i - 4j + 4k, v = 8i + 8j - 8k
A. 0; 4i+ 4j - 4k
B. 12;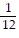 
(4i + 4j - 4k)
C. 4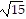
; 
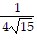(4i + 4j - 4k)
D. 0; no direction
Multiply using the Russian Peasant method.68 × 358
A. 24,024 B. 24,304 C. 24,344 D. 24,334
Classify the triangle as equilateral, isosceles, or scalene. Also, classify the triangle as acute, right, or obtuse.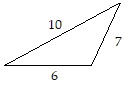
A. isosceles; obtuse B. isosceles; acute C. scalene; obtuse D. scalene; acute
Analyze the graph of the given function f as follows:(a) Determine the end behavior: find the power function that the graph of f resembles for large values of |x|.(b) Find the x- and y-intercepts of the graph.(c) Determine whether the graph crosses or touches the x-axis at each x-intercept.(d) Graph f using a graphing utility.(e) Use the graph to determine the local maxima and local minima, if any exist. Round turning points to two decimal places.(f) Use the information obtained in (a) - (e) to draw a complete graph of f by hand. Label all intercepts and turning points.(g) Find the domain of f. Use the graph to find the range of f.(h) Use the graph to determine where f is increasing and where f is decreasing.f(x) = -2(x - 3)(x + 1)3
What will be an ideal response?