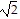Solve the problem.An airplane has an air speed of 550 miles per hour bearing N30°W. The wind velocity is 50 kilometers per hour in the direction N30°E. Find the resultant vector (with exact components) representing the path of the plane relative to the ground. To the nearest tenth, what is the ground speed of the plane? What is its direction?
What will be an ideal response?
-250i + 300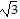
j; 576.6 mph; N25.7°W
You might also like to view...
A ball is tossed upward and then falls to the ground. Its height after t seconds is given by the function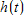 
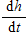
. During the period the rock is fa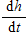lling, what can b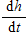e said about 
?
?
A. is positive.
B. is negative.
C. 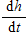is zero.
D. None of the above
Find the extreme values of the function subject to the given constraint.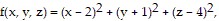
A. Maximum: none; minimum: 
at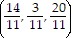 
B. Maximum: none; minimum: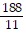 
at 
C. Maximum: none; minimum: 
at 
D. Maximum: none; minimum: 
at 
Divide and/or multiply as indicated. Simplify and leave the numerator and denominator in your answer in factored form.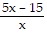
÷ 
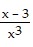
A. 5x2 + 5x
B. 5x2
C.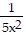 
D. 
Simplify. Assume that all variables represent positive real numbers.6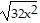
- 2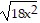
- 
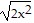
A. 3x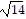
B. 4x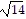
C. 17x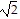
D. 18x