Solve the problem.Use Newton's method to estimate the solutions of the equation 
. Start with
for the right-hand solution and with x0 = -2 for the solution on the left. Then, in each case find x2.
What will be an ideal response?
f(x) = -3x2 - 4x + 1, f'(x) = -6x - 4
Right-hand solution:
x1 = 0.5
xn + 1 = xn -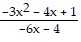 
= 
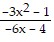
therefore x2 = 0.2500
Left-hand solution:
x1 = -2
xn + 1 = xn -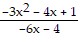 
= 
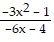
therefore x2 = -1.6250
You might also like to view...
Write the algebraic expression described. Simplify if possible.In the race for Student Body President, Jose received 341 more votes than Angela. If Angela received x votes, how many votes did Jose receive?
A. (341 - x) votes B. (x + 341) votes C. (x - 341) votes D. (341x) votes
Simplify.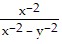
A.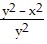 
B.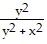 
C. 
D. 
Identify the function's extreme values in the given domain, and say where they are assumed. Tell which of the extreme values, if any, are absolute.f(x) =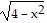 
, -2 ? x < 2
A. local and absolute minimum: 0 at x = -2 and x = 2; local and absolute maximum: 2 at x = 0 B. local and absolute maximum: 0 at x = -2; local and absolute minimum: 2 at x = 0 C. no local extrema; no absolute extrema D. local and absolute minimum: 0 at x = -2; local and absolute maximum: 2 at x = 0
Determine whether the given function is even, odd, or neither.f(x) = x5 - x4
A. Even B. Odd C. Neither