Find the vertex, focus, and directrix of the parabola. Graph the parabola.y2 + 6y = 8x + 39
A. vertex: (-6, -3)
focus: (-6, -1)
directrix: y = -5
B. vertex: (-6, -3)
focus: (-6, -5)
directrix: y = -1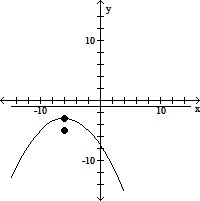
C. vertex: (-6, -3)
focus: (-8, -3)
directrix: x = -4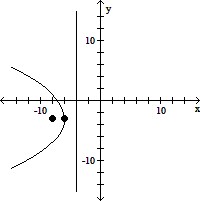
D. vertex: (-6, -3)
focus: (-4, -3)
directrix: x = -8
Answer: D
You might also like to view...
The position vector of a particle is r(t). Find the requested vector.The acceleration at t = 0 for r(t) = t2i + (10t3 - 5)j +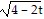 
k
A. a(0) = 2i - 
k
B. a(0) = 2i - 
k
C. a(0) = 2i + 
k
D. a(0) = 2i - 
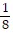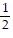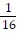k
Identify the curve represented by the equation.x(x + 6y) = 5x2 + 6xy - 5y2 - 4
A. Ellipse B. Hyperbola C. Circle D. None of these
Factor completely.x3y - 5x2y2 - 36xy3
A. x(xy - 9y2)(x + 4y) B. y(x - 9y)(xy + 4y2) C. xy(x - 9y)(x + 4y) D. xy(x2 - 5x - 36y2)
Give the largest interval where the function increases or decreases, as requested.f(x) = x2 + 8; decreases
A. (-8, 0) B. (0, 8) C. (-?, 0) D. (0, ?)