Solve the problem.Suppose that a hair dryer operates on a voltage that can be represented by the relation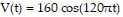 
and that it draws a current represented by the relation 
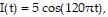where t is time measured in seconds. The power consumed by the appliance is P = VI. Graph the power in 
by 
and use an identity to write the expression for the power in the
form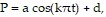 
where a, k, and d are constants.
A. P = 400 cos(120?t) + 400
[0, 0.04, 0.01] by [-200, 2000, 200]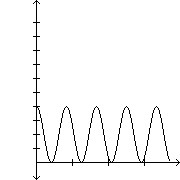
B. P = 800 cos(240?t) + 800
[0, 0.04, 0.01] by [-200, 2000, 200]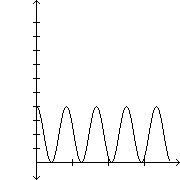
C. P = -400 cos(240?t) + 400
[0, 0.04, 0.01] by [-200, 2000, 200]
D. P = 400 cos(240?t) + 400
[0, 0.04, 0.01] by [-200, 2000, 200]
Answer: D
You might also like to view...
Determine if the series converges or diverges; if the series converges, find its sum.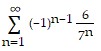
A. Converges; 
B. Converges; 1
C. Converges;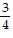 
D. Diverges
Plot the complex number in the complex plane.-2 + 2
i 
A.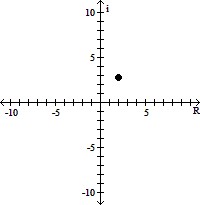 
B. 
C.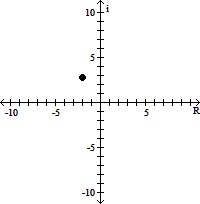 
D. 
Solve the equation. Round any irrational solutions to the nearest thousandth.x3 + x2 + x - 4 = 0
A. 1.151 B. 1.156 C. -1 D. -1.151
Use the leading-term test to match the function with the correct graph.f(x) = -0.7x6 - x5 + 5x4 - 3x3 - 7x2 + x - 3
A.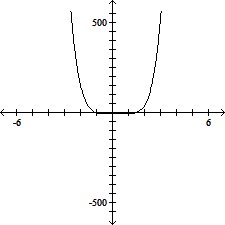 
B.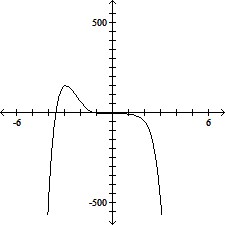 
C.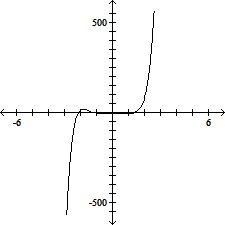 
D. 