Find an upper bound for the magnitude |E| of the error in the linear approximation L to f at the given point over the given region.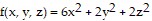
at 
R: |x - 1| ? 0.1, |y + 2| ? 0.1, |z - 3| ? 0.1
A. |E| ? 0.48
B. |E| ? 0.36
C. |E| ? 0.54
D. |E| ? 0.72
Answer: C
Mathematics
You might also like to view...
Approximate the coordinates of each turning point by graphing f(x) in the standard viewing rectangle. Round to the nearest hundredth, if necessary.f(x) = -x3 + 
x2 + 6x - 3
A. (-2, -1) and (1, 3.5) B. (-1, -6.5) and (2, 7) C. (-2, -1) and (-1, -6.5) D. (-1, -6.5) and (1, 3.5)
Mathematics
Factor as completely as possible. If unfactorable, indicate that the polynomial is prime.x4 + 2x3 + 216x + 432
A. (x + 2)(x + 6)(x2 - 6x + 36) B. Prime C. (x + 2)(x3 + 216) D. (x + 2)(x - 6)(x2 + 6x + 36)
Mathematics
Determine whether the test point is a solution to the linear inequality.(-3, -5), y < x - 1
A. Yes B. No
Mathematics
Evaluate: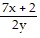 
when x = 4 and y = -5
A. 3 B. - 4 C. 4 D. - 3
Mathematics