Find the vertex, focus, and directrix of the parabola with the given equation.(x - 2)2 = -20(y - 3)
A. vertex: (2, 3)
focus: (2, -2)
directrix: y = 8
B. vertex: (-2, -3)
focus: (-2, -8)
directrix: y = 2
C. vertex: (2, 3)
focus: (2, 8)
directrix: x = -2
D. vertex: (3, 2)
focus: (3, -3)
directrix: y = 7
Answer: A
You might also like to view...
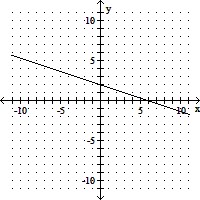
Find the slope of the line.
A. 2 B. -6 C. 0 D. undefined slope
Use a calculator to compute. Round the result to two decimal places.-113.21 - 20.93
A. -134.14 B. -92.28 C. 134.14 D. 92.28
State the instructions of the function in words.f(z) = 6(10z + 5) - 3
A. Multiply the independent variable by 10. Add 5 to this product. Multiply this result by 6. Then subtract 3 from this product. B. Add 5 to the independent variable. Multiply the result by 10. Multiply this result by 6. Then subtract 3 from this product. C. Multiply the independent variable by 6 and then by 10. Add 2 to this result. D. Multiply the independent variable by 10. Add 5 and subtract 3 from this product. Multiply the result by 6.
Find the y- and x-intercepts for the equation. Then graph the equation.2x - 6y = 12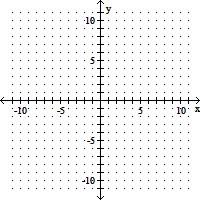
A. (0, -2); (-6, 0)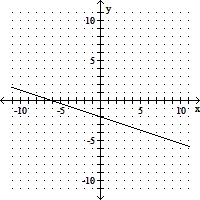
B. (0, 2); (-6, 0)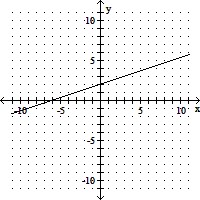
C. (0, -2); (6, 0)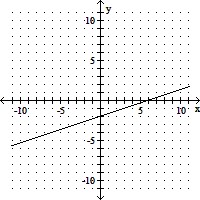
D. (0, 2); (6, 0)