Use synthetic division to find the quotient and the remainder.(2x4 - x3 - 15x2 + 3x) ÷ (x + 3)
A. Q(x) = (2x3 - 5x2 + 3); R(x) = 9
B. Q(x) = (2x3 + 5x2 + 3); R(x) = 9
C. Q(x) = (2x3 - 7x2 + 6x - 15); R(x) = 45
D. Q(x) = (2x3 - 7x2 + 6x - 15); R(x) = - 45
Answer: C
You might also like to view...
Solve the problem.Find the exponential function f that models this data. Round the coefficients to the nearest hundredth.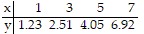
A. f(x) = (0.98)(1.33)x B. f(x) = (1.14)(0.85)x C. f(x) = (0.85)(1.14)x D. f(x) = (1.33)(0.98)x
Determine the maximum possible number of turning points for the graph of the function.f(x) = x2 + 8x3
A. 1 B. 3 C. 8 D. 2
Use the elimination method to solve the system.
A. x = 1, y = 1 B. x = 1, y = 0 C. x = 0, y = 0 D. x = 0, y = 1
Answer the question.What does the digit 7 mean in the number 247,189?
A. 7 hundreds B. 7 thousands C. 7 ten thousands D. 7 hundred thousands