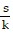Solve the problem.Wildlife management personnel use predator-prey equations to model the populations of certain predators and their prey in the wild. Suppose the population M of a predator after t months is given by M = 750 + 125 sin 
twhile the population N of its primary prey is given by N = 12,250 + 3050 cos 
t.Calculate both M and N for t = 3, t = 15, and t = 27 months. Explain the answers in terms of reference angles. Without calculating them, what will be the values of M and N for t = 39 months?
What will be an ideal response?
For all 3 values of t, M = 875 and N = 12,250;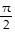 
is the reference angle for the given values of 
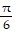t;
for t = 39, M = 875 and N = 12,250.
You might also like to view...
Solve the problem. Use an annual percentage rate table if necessary.A retired couple buys a new recreational vehicle (RV) for $50,000. They make a down payment of $13,000 and finance the balance at 9.0% APR over 60 months. Before making the 30th payment, the couple decides to pay the remaining balance on the loan. How much interest will the couple save (use the actuarial method)?
A. $2447.28 B. $2476.10 C. $3346.09 D. $2774.23
Provide an appropriate response.If Adb = 20 log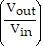 
and Vin = 0.3 V and Vout = 25 V, then Adb =
A. 38.4 db B. -76.8 db C. 76.8 db D. -38.4 db
Write the equation in polar form.x =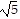 
A. r = 
csc ?
B. r =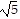 
sec ?
C. r =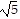 
sin ?
D. r = 
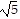cos ?
Write the statement as a power function equation. Use k as the constant of variation.The altitude h of an equilateral triangle varies directly as one side s.
A. h = ks
B. h = 
C. h = ks2
D. h = 