Three factories each dump waste water containing three different types of pollutants into a river. State regulations require the factories to treat their waste in order to reduce pollution levels. The table shows the possible percent reduction of each pollutant at each site and the cost per ton to process the waste.
?
Factory 1
Factory 2
Factory 3
Pollutant 1
75%
45%
65%
Pollutant 2
15%
30%
20%
Pollutant 3
10%
25%
15%
Cost/ton
$52
$21
$8
?
If the state requires a reduction of at least 65 tons per day of pollutant 1, at least 40 tons per day of pollutant 2, and at least 20 tons per day of pollutant 3, find the number of tons of waste that must be treated each day at each site so that the state's requirements are satisfied and the treatment costs are minimized. Find the minimum cost.
?
?
A. The minimum cost is $3,190 per day when Factory #2 and Factory #3 each process 110 tons and Factory #1 does not process any.
B. The minimum cost is $2,920 per day when Factory #1 and Factory #2 each process 40 tons and Factory #3 does not process any.
C. The minimum cost is $1,600 per day when Factory #3 process 200 tons, Factory #1 a and Factory #2 do not process any.
D. The minimum cost is $1,540 per day when Factory #1 and Factory #2 each process 20 tons and Factory #3 produces 10 tons.
E. The minimum cost is $2,490 per day when Factory #2 and Factory #3 each process 50 tons and Factory #1 produces 20 tons.
Answer: C
You might also like to view...
Solve.Each month a beauty salon gives x manicures for $12.00/manicure. The cost to the owner of the beauty salon for each manicure is $7.35. The monthly fixed cost to maintain a manicure station is $120.00. Write an equation that relates the monthly profit, in dollars, to the number of manicures given each month. Then use the equation to find the monthly profit when 200 manicures are given in a month.
A. P = 7.35x - 120; $1350 B. P = 4.65x - 120; $810 C. P = 4.65x; $930 D. P =12x - 120; $2280
Solve the problem.The following points form a quadratic relationship: (1, 5.0), (2, 4.4), (3, 4.3), (4, 4.2), (5, 4.6), (6, 4.8), (7, 5.4), (8, 6.2) . The x-coordinates are the years a particular company has been in operation and the y-coordinates are the profit, in millions, for that year. Find the quadratic function that models the profit in millions as a function of x, the number of years of operation. Round to three decimal places.
A. y = - 0.003x2 + 0.145x - 0.892 B. y = 0.101x2 - 0.723x + 5.552 C. y = 0.099x2 + 1.254x - 3.512 D. y = 0.203x2 - 0.945x + 7.098
Solve the problem.One side of a parallelogram is  and another side is
and another side is  The longer diagonal is
The longer diagonal is  Find the angle opposite the longer diagonal to the nearest tenth of a degree.
Find the angle opposite the longer diagonal to the nearest tenth of a degree.
A. 56.1° B. 31.4° C. 93.2° D. 61.6°
Solve. Write the answer in simplest form.The circle graph shows the fraction of books read by grades one through five. What fraction of books was NOT read by the second and fifth grades?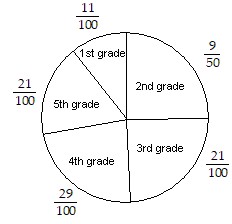
A. 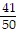
B. 
C. 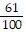
D. 