Use the Principle of Mathematical Induction to show that the statement is true for all natural numbers n.7 + 14 + 21 + ... + 7n =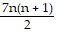 
What will be an ideal response?
First, we show the statement is true when n = 1.
For n = 1, we get 7 =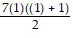 
= 7.
This is a true statement and Condition I is satisfied.
Next, we assume the statement holds for some k. That is,
is true for some positive integer k.
We need to show that the statement holds for k + 1. That is, we need to show that
.
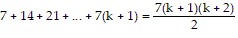So we assume that 
is true and add the next term,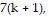 
to both sides of the equation.
Condition II is satisfied. As a result, the statement is true for all natural numbers n.
You might also like to view...
Perform the indicated operation. Write the answer in lowest terms.2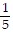
÷ 1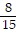
A. 2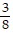
B. 1
C. 3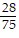
D. 1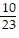
The graph of the given function is drawn with a solid line. The graph of a function, g(x), transformed from this one is drawn with a dashed line. Find a formula for g(x).f(x) = x3
A. g(x) = (x + 2)3 B. g(x) = 2x3 C. g(x) = (x - 2)3 D. g(x) = x3 + 2
Evaluate the exponential expression.(-2)2
A. -4 B. -8 C. 4 D. 2
Write an equivalent expression. Use an associative law.(9s)t
A. 9(st) B. (s9)t C. 9s + 9t D. t(9s)