Find the center, transverse axis, vertices, and foci of the hyperbola.
- 
= 1
A. center at (0, 0)
transverse axis is y-axis
vertices: (-7, 0), (7, 0)
foci: (-5, 0), (5, 0)
B. center at (0, 0)
transverse axis is y-axis
vertices: (0, -7), (0, 7)
foci: (- 
, 0), (
, 0)
C. center at (0, 0)
transverse axis is x-axis
vertices: (-5, 0), (5, 0)
foci: (- 
, 0), (
, 0)
D. center at (0, 0)
transverse axis is y-axis
vertices: (0, -7), (0, 7)
foci: (0, - 
), (0, 
)
Answer: D
You might also like to view...
Use the quadratic formula to solve the equation. Simplify any radicals.3k2 - 11k - 4 = 0
A. {-3, 4}
B. 
C.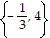 
D.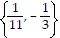 
Evaluate.(-1)13
A. 1 B. -13 C. -1 D. 13
Find the equation of the parabola determined by the given information.Vertex at (4, 4), directrix x = 2
A. x = 
y2 - 1y + 6
B. y = 
x2 - 6x - 24
C. x = 
y2 + 8y + 48
D. y =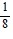 
x2 - 1x - 6
Perform the indicated operations.(-3x2 - 7) - (-x3 + 9x2 - 5)
A. -2x3 + 9x2 - 2 B. x3 + 6x2 - 12 C. -2x3 + 2x2 + 5 D. x3 - 12x2 - 2