Use calculus to find any critical points and inflection points of the given function. Then determine the concavity of the function and the intervals over which it is increasing/decreasing.f(x) = e-(1/4)x
A. Critical points: critical point at x = 0
Inflection points: none
Concavity: concave up for all real numbers
Increasing: increasing for all x < 0 and decreasing for all x > 0
B. Critical points: none
Inflection points: point of inflection at x = 0
Concavity: concave down for all x < 0 and concave up for all x > 0
Decreasing: decreasing for all real numbers
C. Critical points: none
Inflection points: none
Concavity: concave up for all real numbers
Decreasing: decreasing for all real numbers
D. Critical points: none
Inflection points: none
Concavity: concave down for all real numbers
Decreasing: decreasing for all real numbers
Answer: C
You might also like to view...
Perform the indicated operation and round as indicated. hundredths
hundredths
A. 0.72 B. 0.02 C. 0.07 D. 0.22
Round as indicated.548,039 to the nearest ten
A. 548,020 B. 548,040 C. 548,050 D. 548,140
Graph f by hand by first plotting points to determine the shape of the graph.f(x) = -  x2
x2
A. 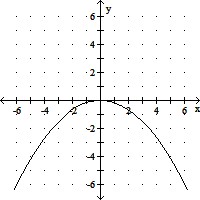
B. 
C. 
D. 
Solve the equation.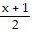 =
= 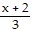
A. x = -1 B. x = 1 C. x = -3 D. x = 3