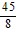Find the center, transverse axis, vertices, foci, and the equations of the asymptotes of the hyperbola.(x + 1)2 - 9(y - 4)2 = 9
A. center: (-1, 4)
transverse axis: y = 4
vertices: (-2, 4) and (0, 4)
foci: (-1 - 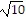 , 4) and (-1 +
, 4) and (-1 + 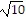 , 4)
, 4)
asymptotes: y - 4 = - 3(x + 1) and y - 4 = 3(x + 1)
B. center: (4, -1)
transverse axis: y = 4
vertices: (1, -1) and (7, -1)
foci: (4 - 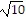 , -1) and (4 +
, -1) and (4 + 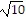 , -1)
, -1)
asymptotes: y + 1 = -  (x - 4) and y + 1 =
(x - 4) and y + 1 = 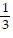 (x - 4)
(x - 4)
C. center: (-1, 4)
transverse axis: x = -1
vertices: (-1, 1) and (-1, 7),
foci: (-1, 4 -  ) and (-1, 4 +
) and (-1, 4 +  ),
),
asymptotes: y + 4 = - 3(x - 1) and y + 4 = 3(x - 1)
D. center: (-1, 4)
transverse axis: y = 4
vertices: (-4, 4) and (2, 4)
foci: (-1 -  , 4) and (-1 +
, 4) and (-1 + 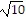 , 4)
, 4)
asymptotes: y - 4 = - 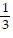 (x + 1) and y - 4 =
(x + 1) and y - 4 = 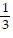 (x + 1)
(x + 1)
Answer: D
You might also like to view...
Perform the indicated operation. Write the result in standard form.(9 + 8i) - (-8 - 8i) - (-4 - 2i)
A. 21 - 2i B. 21 + 18i C. -3 - 2i D. 13 + 14i
Solve the equation.43 - x = 24 (Round to the nearest hundredth.)
A. 3.00 B. -3.00 C. 0.71 D. 3.44
Sketch the graph of the quadratic function. Identify the vertex and axis of symmetry.f(x) = (x - 11)2
A. vertex: (11, 0)
axis of symmetry: x = 11
B. vertex: (0, 0)
axis of symmetry: x = 0
C. vertex: (0, -11)
axis of symmetry: x = 0
D. vertex: (-11, 0)
axis of symmetry: x = -11
Solve the equation. Give an exact solution.ln(eln(x)) - ln(x - 5) = ln(9)
A. 5, 9
B. - 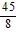 ,
, 
C. No solution
D.