Solve.The manager of a store determines that the store can sell x items at a price of 125 - 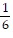 x dollars per item. (i) Write a formula for the total revenue R that these sales generate.(ii) Using the formula in part (i), complete a table of the revenue generated by the sale of 0, 100, 200, 300 and 400 items.x0100200300400R?????(iii) Graph the formula.(iv) How many items should the store sell to maximize the revenue?(v) What is the maximum revenue?
x dollars per item. (i) Write a formula for the total revenue R that these sales generate.(ii) Using the formula in part (i), complete a table of the revenue generated by the sale of 0, 100, 200, 300 and 400 items.x0100200300400R?????(iii) Graph the formula.(iv) How many items should the store sell to maximize the revenue?(v) What is the maximum revenue?
What will be an ideal response?
(i) R = x = 125x -
= 125x -  x2
x2
(ii)
| x | 0 | 100 | 200 | 300 | 400 |
| R | 0 | 10,833.33? | 18,333.33? | 22,500? | 23,333.33? |
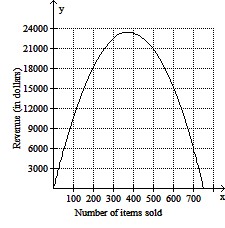
(iv) The store should sell 375 items to maximize the revenue.
(v) The maximum revenue is $23,437.50.
You might also like to view...
Solve the problem.At time 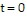 a particle is located at the point (1, 1, 1). It travels in a straight line to the point
a particle is located at the point (1, 1, 1). It travels in a straight line to the point  has speed 2 at (1, 1, 1) and constant acceleration
has speed 2 at (1, 1, 1) and constant acceleration 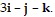 Find an equation for the position vector r(t) of the particle at time t.
Find an equation for the position vector r(t) of the particle at time t.
A. r(t) = 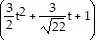 i -
i - 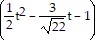 j -
j -  k
k
B. r(t) = 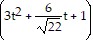 i -
i - 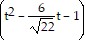 j -
j - 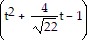 k
k
C. r(t) = 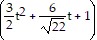 i -
i -  j -
j - 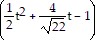 k
k
D. r(t) = 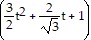 i -
i -  j -
j - 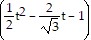 k
k
Use the Consumer Price Index below to answer the question.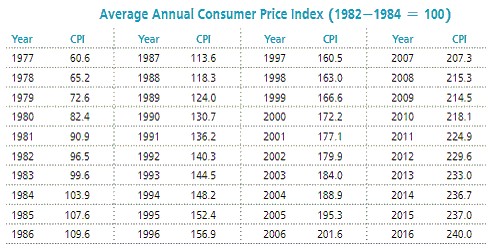 Suppose you needed $48,000 to maintain a particular standard of living in 1986. How much would you have needed in 1994 to maintain the same standard of living? If necessary, round values to the nearest thousandth.
Suppose you needed $48,000 to maintain a particular standard of living in 1986. How much would you have needed in 1994 to maintain the same standard of living? If necessary, round values to the nearest thousandth.
A. $60,000 B. $65,000 C. $68,000 D. $35,000
Rationalize the denominator of the expression.
?
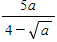
What will be an ideal response?
Use the given graph to find the x-intercepts and zeros of the function.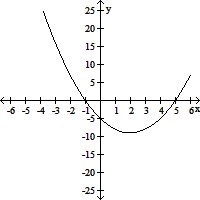
A. (-5, 0), (-1, 0); -5, -1 B. (5, 0), (1, 0); 5, 1 C. (-5, 0), (1, 0); -5, 1 D. (-1, 0), (5, 0); -1, 5