Solve the problem.A population of algae consists of 4000 algae at time t = 0. Conditions will support at most 500,000 algae. Assume that the rate of growth of algae is proportional both to the number present (in thousands) and to the difference between 500,000 and the number present (in thousands). Write a differential equation using 0.01 for the constant of proportionality.
A. dy/dt = 4000y(500 - 0.01y)
B. dy/dt = 0.01(500 - y)
C. dy/dt = 0.01y(y - 500)
D. dy/dt = 0.01y(500 - y)
Answer: D
You might also like to view...
Find the orthogonal trajectories of the family of curves. Sketch several members of each family.y = -mx
What will be an ideal response?
Determine if the statement is a proportion.I. 

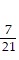 II.
II. 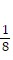


A. I is a proportion, II is not a proportion. B. I is not a proportion, II is a proportion. C. I and II are both proportions. D. Neither I nor II are proportions.
Let f be the function defined by 

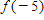
class="wirisformula" align="middle" style="vertical-align: middle;" data-wiris-created="true" varid="variable_id_field" variablename="impvar_49d4a7398c74433c9b60642af" />, 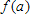

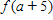
What will be an ideal response?
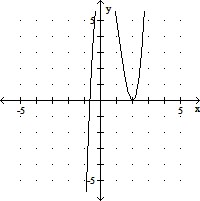
Graph the polynomial function. Factor first if the expression is not in factored form.f(x) = (-3x - 2)(x - 2)2
A. 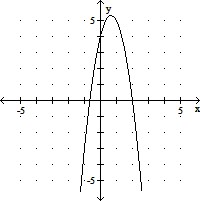
B. 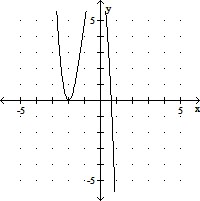
C. 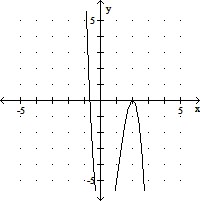
D.