Solve the problem.Halley's comet has an elliptical orbit with the sun at one focus. Its orbit shown below is given approximately by 
In the formula, r is measured in astronomical units. (One astronomical unit is the average distance from Earth to the sun, approximately 93 million miles.) 
Find the distance from Halley's comet to the sun at its shortest distance from the sun. Round to the nearest hundredth of an astronomical unit and the nearest million miles.
A. 5.36 astronomical units; 499 million miles
B. 585 astronomical units; 54,405 million miles
C. 5.31 astronomical units; 494 million miles
D. 10.72 astronomical units; 997 million miles
Answer: C
You might also like to view...
Evaluate the polynomial for the given value.A rocket is stopped 26 feet from a satellite when it begins accelerating away from the satellite at a constant rate of 10 feet per second per second. The distance between the rocket and the satellite is given by the polynomial 5t2 + 26. Find the distance between the rocket and the satellite 11 seconds after the rocket started moving.
A. 631 ft B. 605 ft C. 147 ft D. 81 ft
Find the exact value by using a sum or difference identity.cos 345°
A.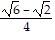 
B.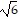 
- 
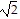
C.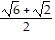 
D.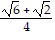 
Factor the square of the binomial.a3 + 14a2 + 49a
A. a(a - 1)(a + 49) B. (a2 + 7)(a - 7) C. a(a + 7)(a - 7) D. a(a + 7)2
Perform the indicated matrix operations.Let A = 
and B = 
. Find 2A + 2B.
A.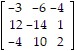 
B. 
C. 
D. 