Solve the problem.A steel can in the shape of a right circular cylinder must be designed to hold 550 cubic centimeters of juice (see figure). It can be shown that the total surface area of the can (including the ends) is given by S(r) = 2?r2 +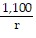 
, where r is the radius of the can in centimeters. Using the TABLE feature of a graphing utility, find the radius that minimizes the surface area (and thus the cost) of the can. Round to the nearest tenth of a centimeter. 
A. 0 cm
B. 3.6 cm
C. 4.4 cm
D. 5.6 cm
Answer: C
Mathematics
You might also like to view...
Graph the function.f(x) = 
A.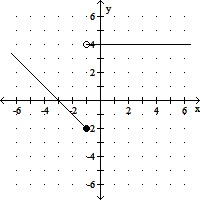 
B.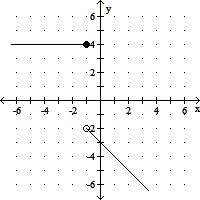 
C. 
D. 
Mathematics
Answer the question.List all numbers that must be rejected as possible solutions.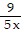
- 
= 
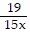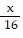
A. There are no numbers that must be rejected. B. 5, 15 C. 5, 15, 16 D. 0
Mathematics
Provide an appropriate response.Which one of the following is a description of the graph of the solution set for the given system?4x2 + 9y2 < 36y < -x
A. Outside the ellipse and below the line B. Inside the ellipse and below the line C. Inside the ellipse and above the line D. Outside the ellipse and above the line
Mathematics
Sketch the graph of the function.f(x) =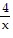 
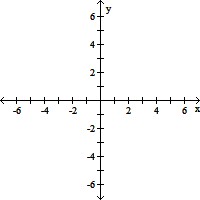
A.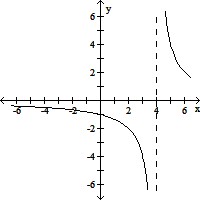 
B. 
C.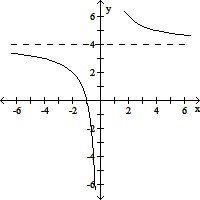 
D.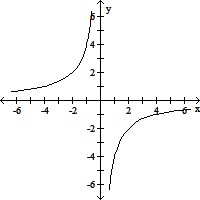 
Mathematics