Solve the problem.Suppose the following matrix represents the input-output matrix of a primitive economy that depends on two basic goods, yams and pigs. How much of each commodity should be produced to satisfy a demand for 63 bushels of yams and 52 pigs? (It is not necessary to round to a whole-number quantity of pigs.)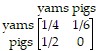
A. 107.5 bushels of yams and 105.75 pigs
B. 153 bushels of yams and 74.25 pigs
C. 107.5 bushels of yams and 129.38 pigs
D. 133.5 bushels of yams and 74.25 pigs
Answer: A
You might also like to view...
The given pattern continues. Write down the nth term of the sequence suggested by the pattern.-3, 9, -27, 81, . . .
A. an = (-1)n+1 3n+1 B. an = (-1)n 3n C. an = 3n+1 D. an = 3n
Use the given vectors to find the indicated expression.v = -2i - 5j - 4k, w = 3i - 4j + 2kFind v × (5w).
A. -66i + 8j + 55k B. -90i - 56j + 83k C. 130i + 10j - 115k D. -130i - 40j + 115k
Complete the identity.sin2 x + tan2 x + cos2 x = ?
A. tan2 x B. cot3 x C. sin x D. sec2 x
Use algebraic and/or graphical methods to solve the inequality.(x + 7)(x + 6)(x - 5) < 0
A. x < -6 B. -7 < x < -6 or x > 5 C. x > 5 D. x < -7 or -6 < x < 5