Graph the function by hand, not by plotting points, but by starting with the graph of one of the standard functions and then applying the appropriate transformations.
 multiple choice.png)
 ANSWER.png)
You might also like to view...
Use Euler's method to calculate the first three approximations to the given initial value problem for the specified increment size. Round your results to four decimal places.y' = 4xex4, y(1) = 2, dx = 0.1
A. y1 = 3.0873, y2 = 4.9897, y3 = 8.8074 B. y1 = 2.4699, y2 = 4.3723, y3 = 8.1899 C. y1 = 2.7786, y2 = 4.6810, y3 = 8.4986 D. y1 = 3.3960, y2 = 5.2985, y3 = 9.1161
Provide an appropriate response.Do the points (0, 0), (1, -5) and (-1, 5) line on the same line? Explain.
A. Yes, the points do lie on the same line. The line containing (0, 0) and (1, -5) is y = 5x. The line containing (0, 0) and (-1, 5) is also y = 5x. B. No, the points do not lie on the same line. The line containing (0, 0) and (1, -5) is y = -5x. The line containing (0, 0) and (-1, 5) is y = 5x. C. No, the points do not lie on the same line. The line containing (0, 0) and (1, -5) is y = 5x. The line containing (0, 0) and (-1, 5) is y = -5x. D. Yes, the points do lie on the same line. The line containing (0, 0) and (1, -5) is y = -5x. The line containing (0, 0) and (-1, 5) is also y = -5x.
Decide if the given equation is a solution of the given differential equation.
= 2
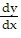, y = 2e2x - 5
A. No B. Yes
Find the values of x for which the equation is true.
- 5 = 7
A. -12 B. 12 C. 2, -2 D. 12, -12