Calculate dy/dt using the given information.x4/3 + y4/3 = 2; dx/dt = 6, x = 1, y = 1
A. 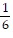
B. - 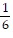
C. 6
D. -6
Answer: D
You might also like to view...
Evaluate the expression by hand.64-4/3
A. 16,384
B. 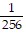
C. 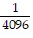
D. 1024
Solve the problem.Atomic nuclei consist of protons and neutrons. The number of protons and neutrons in a particular nucleus must fall into a well-defined range of values in order for the nucleus to be stable, else it will be unstable and will disintegrate by radioactive decay. The figure below shows this so-called "region of stability" for nuclei having proton numbers between 10 and 60. Determine a system of linear inequalities that describe the region of stability. Let x represent the number of protons and y represent the number of neutrons.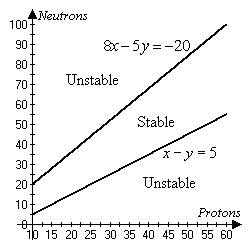
A. y ? x - 5
y ? 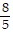 x + 4
x + 4
B. y ? x - 5
y ? 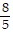 x + 4
x + 4
C. y ? x - 5
y ? 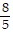 x + 4
x + 4
D. y ? x - 5
y ? 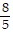 x + 4
x + 4
Form a polynomial whose zeros and degree are given. Use a leading coefficient of 1. Zeros: -3, -6, 6; degree 3
A. f(x) = x3 + 36x + 3x2 + 108 B. f(x) = x3 + 36x - 3x2 - 108 C. f(x) = x3 - 36x + 3x2 - 108 D. f(x) = x3 - 36x - 3x2 + 108
Find all points where the function has any relative extrema or saddle points and identify the type of relative extremum.f(x, y) = ex+y
A. Saddle point at (0, 0) B. Relative minimum at (0, 0) C. Relative minimum at (0, 1) D. No relative extrema.