Solve the problem.A ball is dropped from a height of 25 feet. Each time it strikes the ground, it bounces up to 0.7 of the previous height. The total distance the ball has travelled before the second bounce is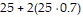 
feet, and the total distance the ball has travelled before bounce n + 1 is 25 + 
feet.Use facts about infinite geometric series to calculate the total distance the ball has travelled by the time it has stopped bouncing.
Fill in the blank(s) with the appropriate word(s).
141(2/3) feet
You might also like to view...
Graph the quadratic function. Determine the vertex, find the equation of the axis of symmetry, find any x- and y-intercepts, and state the domain and range in interval notation.f(x) = x2 + 6x + 5
A. vertex: (3, 4)
axis of symmetry: x = 3
x-intercepts: 1 and 5; y-intercept: -5
domain: (-?, ?); range: (-?, 4]
B. vertex: (-3, 4)
axis of symmetry: x = -3
x-intercepts: -1 and - 5; y-intercept: -5
domain: (-?, ?); range: (-?, 4]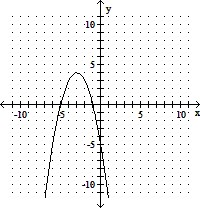
C. vertex: (-3, -4)
axis of symmetry: x = -3
x-intercepts: -1 and - 5; y-intercept: 5
domain: (-?, ?); range: [-4, ?]
D. vertex: (3, -4)
axis of symmetry: x = 3
x-intercepts: 1 and 5; y-intercept: 5
domain: (-?, ?); range: [-4, ?]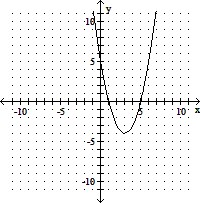
Solve the absolute value equation.|4x - 8| = |x + 2|
A.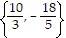 
B.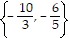 
C.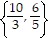 
D. ?
Solve the inequality.x2 - 9x + 20 > 0
A. x < 4 or x > 5 B. x < 4 C. 4 < x < 5 D. x > 5
Find the slope of the line. Interpret the slope in terms of rise and run.
A. Undefined; The run always equals zero B. 0; The rise always equals zero C. 2; The graph rises 2 units for each unit of run. D. -1; The graph falls 1 unit for each unit of run.