If an argument reads
If ?ABC is an equilateral triangle with perimeter 3s units then each edge of ABC is s units long.
If each edge of an equilateral triangle is s units long then its height is
 units
units
If the height of an equilateral triangle is
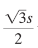
units, then its area is
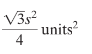
Then we can deduce
(a) If ABC is an equilateral triangle with perimeter 3s units then its area is
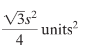
(b) If the area of ABC is
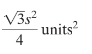
then it is an equilateral triangle
(c) If each edge of an equilateral triangle is s units long then its area is

(d) All of these are valid conclusions.
(e) Both (a) and (c) are correct.
(e) Both (a) and (c) are correct.
You might also like to view...
Specify the domain of the function.f(x) = 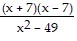
A. x ? 49 B. x ? 7, x ? -7 C. All real numbers D. x > 49
Solve the problem.Find two numbers whose sum is 27 and whose difference is 1.
A. 14 and 13 B. 15 and 16 C. 12 and 15 D. 27 and 0
Write the radical expression as an exponential expression.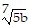
A. 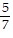 b
b
B. (5b)5/7
C. (5b)1/7
D. 5b1/7
Plot the point.(-2, 6)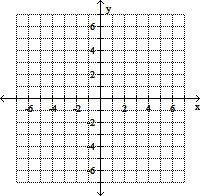
A. 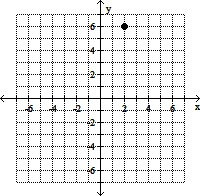
B. 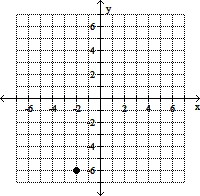
C. 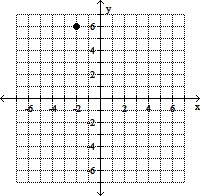
D. 