Find a matrix A and a column matrix B that describe the following tables involving credits and tuition costs. Find the matrix product AB, and interpret the significance of the entries of this product.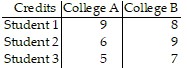
?
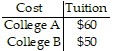
A.
AB = 
Tuition for Student 1 is $940, tuition for Student 2 is $810, and tuition for Student 3 is $650.
B.
AB = 
The number of credits for Student 1 is 12, the number of credits for Student 2 is 15, and the number of credits for Student 3 is 12.
C.
AB =
Tuition for Student 1 is $840, tuition for Student 2 is $660, and tuition for Student 3 is $900.
D.
AB = 
The number of credits for Student 1 is 12, the number of credits for Student 2 is 17, and the number of credits for Student 3 is 15.
Answer: A
You might also like to view...
Find the Taylor series generated by f at x = a.f(x) = x4 - 3x2 - 3x - 9, a = -2
A. (x + 2)4 + 8(x + 2)3 + 21(x + 2)2 - 17(x + 2) - 43 B. (x + 2)4 - 8(x + 2)3 + 21(x + 2)2 - 23(x + 2) + 1 C. (x + 2)4 - 8(x + 2)3 + 21(x + 2)2 - 17(x + 2) - 43 D. (x + 2)4 + 8(x + 2)3 + 21(x + 2)2 - 23(x + 2) + 1
Write a polar equation of the conic that has a focus at the origin, eccentricity  FIGURE 1.png)
, and directrix y = -7. Identify the conic.
What will be an ideal response?
Find the amount of sales tax or tax rate and the total cost. Round money answers to the nearest cent if necessary.Amount of sale: $870 Tax rate: 7%
A. $69.60, $939.60 B. $52.20, $922.20 C. $60.90, $930.90 D. $609.00, $1479.00
Factor.t3 + 125
A. (t - 5)(t2 + 5t + 25) B. (t - 125)(t2 - 1) C. (t + 5)(t2 + 25) D. (t + 5)(t2 - 5t + 25)