Solve the problem.Find a matrix A and a column matrix B that describe the following tables involving credits and tuition costs. Find the matrix product AB and interpret the significance of the entries of this product.

A.
AB = 
Tuition for Student 1 is $1164 and tuition for Student 2 is $885.
B.
AB = 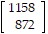
Tuition for Student 2 is $1158 and tuition for Student 1 is $872.
C.
AB = 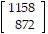
Tuition for Student 1 is $1158 and tuition for Student 2 is $872.
D.
AB = 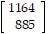
Tuition for Student 2 is $1164 and tuition for Student 1 is $885.
Answer: A
You might also like to view...
Use a proportion to solve the problem.Find the length, L, of the lake. Assume that the two triangles are similar. 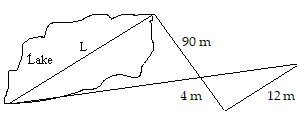
A. 450 m B. 30 m C. 8100 m D. 270 m
Solve each problem.Find two numbers whose sum is 450 and whose product is as large as possible.
A. 10 and 440 B. 224 and 226 C. 225 and 225 D. 1 and 449
diagnostic test for a certain disease has a sensitivity of 0.92 and a specificity of 0.88. The probability that a patient has the disease is estimated as 0.1. The diagnostic test is given and returns positive. What is the posterior probability that the patient has the disease?
What will be an ideal response?
Solve.Two rectangles each have an area of 32 ft2. One rectangle has a width 4 ft greater and a length 4 ft shorter than the other rectangle. Find the shorter width.
A. 5 ft B. 4 ft C. 8 ft D. 7 ft