State the power and constant of variation for the function, and then analyze it.f(x) = 2x-3
What will be an ideal response?
Power is -3; constant of variation is 2; Domain: (-?, 0) ? (0, ?); Range: (-?, 0) ? (0, ?); Continuous on its domain. Discontinuous at x = 0; Decreasing for all x; Symmetric with respect to the origin (an odd function); Not bounded above or below; No local extrema; Horizontal asymptote: y = 0. Vertical asymptote: x = 0; End behavior: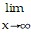 
(2x-3) = 0 and 
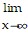(2x-3) = 0.
You might also like to view...
Find the limit of the sequence or determine that the limit does not exist.an = 
n
A. 1 B. does not exist C. e-8 D. e
Use the Remainder Theorem to find the remainder.f(x) = -2x2 - 6x - 6 is divided by x -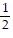 
A.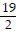 
B. -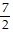 
C. -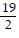 
D.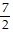 
Add.1 + (-3)
A. 4 B. -2 C. 2 D. -4
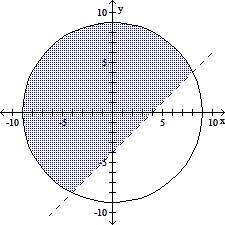
Graph the system of inequalities. Shade the region that represents the solution set.x2 + y2 ? 81x + y > 4
A.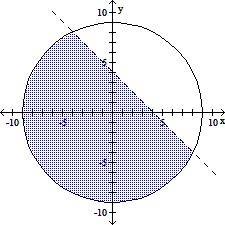 
B. 
C. 
D. 