Evaluate the algebraic expression for the given value or values of the variable(s).5x + 3;
x = 3
A. 8
B. 12
C. 18
D. 30
Answer: C
You might also like to view...
Solve the problem.The mathematical model C = 500x + 60,000 represents the cost in dollars a company has in manufacturing x items during a month. Based on this model, how much does it cost to produce 700 items?
A. $350,000
B. $
C. $120
D. $410,000
Provide an appropriate response.The Consumer Price Index is increasing at a rate of 8% per year. What is its doubling time? Use the approximate doubling time formula (rule of 70).
A. 256 years B. 5.6 years C. 16 years D. 8.75 years
Solve the problem.A space heater can raise the temperature in a room by 2°F every 5 minutes. The temperature in the room was 69°F after the heater had been running for 10 minutes. (i) Write as a linear equation in slope-intercept form the relationship between the time that the heater has been running and the temperature in the room.(ii) Explain how you could have predicted whether the slope of the graph of this equation is positive or negative.
A. (i) y =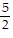 
x - 65
(ii) As the amount of time the space heater is left on increases, the temperature of the room increases, which implies a positive slope.
B. (i) y =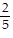 
x + 65
(ii) As the amount of time the space heater is left on increases, the temperature of the room increases, which implies a positive slope.
C. (i) y = 
x + 65
(ii) As the amount of time the space heater is left on increases, the temperature of the room increases, which implies a positive slope.
D. (i) y =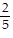 
x - 65
(ii) As the amount of time the space heater is left on increases, the temperature of the room increases, which implies a positive slope.
Find an equation of the parabola described and state the two points that define the latus rectum.Focus at (0, 3); directrix the line y = -3
A. y2 = 16x; latus rectum: (7, 8) and (-7, 8) B. x2 = 12y; latus rectum: (6, 3) and (-6, 3) C. x2 = 12y; latus rectum: (3, 6) and (-3, 6) D. x2 = 16y; latus rectum: (8, 3) and (-8, 3)