Suppose that people's heights (in centimeters) are normally distributed, with a mean of 170 and a standard deviation of 5. We find the heights of 50 people. How many would you expect to be between 160 and 170 cm? Round your answer to the nearest number.
Standard Normal Distribution; z-scores
z 0.00 0.01 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.090.0 0.0000 0.0040 0.0080 0.0120 0.0160 0.0199 0.0239 0.0279 0.0319 0.03590.1 0.0398 0.0438 0.0478 0.0517 0.0557 0.0596 0.0636 0.0675 0.0714 0.07530.2 0.0793 0.0832 0.0871 0.0910 0.0948 0.0987 0.1026 0.1064 0.1103 0.11410.3 0.1179 0.1217 0.1255 0.1293 0.1331 0.1368 0.1406 0.1443 0.1480 0.15170.4 0.1554 0.1591 0.1628 0.1664 0.1700 0.1736 0.1772 0.1808 0.1844 0.18790.5 0.1915 0.1950 0.1985 0.2019 0.2054 0.2088 0.2123 0.2157 0.2190 0.22240.6 0.2257 0.2291 0.2324 0.2357 0.2389 0.2422 0.2454 0.2486 0.2517 0.25490.7 0.2580 0.2611 0.2642 0.2673 0.2704 0.2734 0.2764 0.2794 0.2823 0.28520.8 0.2881 0.2910 0.2939 0.2967 0.2995 0.3023 0.3051 0.3078 0.3106 0.31330.9 0.3159 0.3186 0.3212 0.3238 0.3264 0.3289 0.3315 0.3340 0.3365 0.33891.0 0.3413 0.3438 0.3461 0.3485 0.3508 0.3531 0.3554 0.3577 0.3599 0.36211.1 0.3643 0.3665 0.3686 0.3708 0.3729 0.3749 0.3770 0.3790 0.3810 0.38301.2 0.3849 0.3869 0.3888 0.3907 0.3925 0.3944 0.3962 0.3980 0.3997 0.40151.3 0.4032 0.4049 0.4066 0.4082 0.4099 0.4115 0.4131 0.4147 0.4162 0.41771.4 0.4192 0.4207 0.4222 0.4236 0.4251 0.4265 0.4279 0.4292 0.4306 0.43191.5 0.4332 0.4345 0.4357 0.4370 0.4382 0.4394 0.4406 0.4418 0.4429 0.44411.6 0.4452 0.4463 0.4474 0.4484 0.4495 0.4505 0.4515 0.4525 0.4535 0.45451.7 0.4554 0.4564 0.4573 0.4582 0.4591 0.4599 0.4608 0.4616 0.4625 0.46331.8 0.4641 0.4649 0.4656 0.4664 0.4671 0.4678 0.4686 0.4692 0.4699 0.47061.9 0.4713 0.4719 0.4726 0.4732 0.4738 0.4744 0.4750 0.4756 0.4761 0.47672.0 0.4772 0.4778 0.4783 0.4788 0.4793 0.4798 0.4803 0.4808 0.4812 0.48173.0 0.4987 0.4987 0.4987 0.4988 0.4988 0.4989 0.4989 0.4989 0.4990 0.4990?
__________ people
Fill in the blank(s) with the appropriate word(s).
24
You might also like to view...
Part of $3,800 is invested at 12%, another part at 13%, and the remainder at 14%. The total yearly income from the three investments is $506. The sum of the amounts invested at 12% and 13% equals the amount invested at 14%. Determine how much is invested at each rate.
A. $1,100 at 12%, $800 at 13%, $1,900 at 14% B. $700 at 12%, $1,200 at 13%, $1,900 at 14% C. $700 at 12%, $1,900 at 13%, $1,200 at 14% D. $600 at 12%, $1,300 at 13%, $1,900 at 14% E. $800 at 12%, $1,300 at 13%, $1,700 at 14%
Graph the inverse of the function plotted, on the same set of axes. Use a dashed curve for the inverse.
A.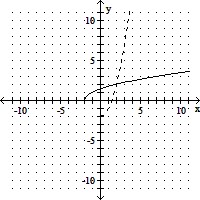 
B. 
C.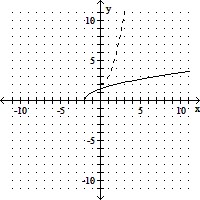 
D. 
Solve the problem.The function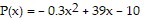 
describes a company's net monthly profit or loss and the function 
describes its monthly costs, where x represents the number of units produced. The total revenue function, R(x), is such that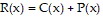 
. Find R(x).
A. R(x) = 50x - 0.3x2 B. R(x) = 49x - 0.6x2 C. R(x) = 52x - 0.3x2 D. R(x) = 50x + 0.3x2
Change the fraction or mixed number into an equivalent decimal. Use bar notation to write repeating decimals.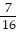 
A. 0.4375 B. 0.4475 C. 0.3375 D. 0.438