How many four-digit numbers can be formed under the following condition?
The leading digit cannot be zero and the number must be less than 7,000.
a. 5,800
b. 6,000
c. 5,700
d. 5,900
b. 6,000
There are 6,000 possible four-digit numbers that can be formed if the leading digit cannot be zero and the number must be less than 7,000.
You might also like to view...
Solve the problem.Find the values of v0 in the equation e =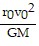 
- 1 that make the orbit described by 
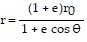parabolic.
A. v0 = 2GM
B. v0 =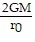 
C. v0 =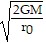 
D. v0 =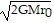 
Determine which fractions are proper.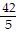
, 
, 
, 
, 
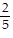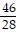A.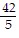 
, 
, 
, 
B. 
, 
, 
, 
, 
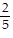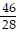C. 
, 
D.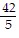 
, 
, 
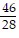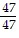
Solve the problem.A pension fund invests $114,200 in municipal bonds and earns 13% per year on the investment. How much money is earned per year?
A. $14,846 B. $878,462 C. $87,846 D. $148,460
Answer the question or provide an example or explanation.Give an example of two binomials whose product is a binomial.
What will be an ideal response?