Complete the table.InputOutput11 ÷ 4 - 0.25 = 22 ÷ 4 - 0.25 = 33 ÷ 4 - 0.25 = 44 ÷ 4 - 0.25 =
A.
| Input | Output |
| 1 | 1 ÷ 4 - 0.25 = 0 |
| 2 | 2 ÷ 4 - 0.25 = 0.25 |
| 3 | 3 ÷ 4 - 0.25 = 0.5 |
| 4 | 4 ÷ 4 - 0.25 = 0.75 |
B.
| Input | Output |
| 1 | 1 ÷ 4 - 0.25 = 0 |
| 2 | 2 ÷ 4 - 0.25 = 0.5 |
| 3 | 3 ÷ 4 - 0.25 = 1 |
| 4 | 4 ÷ 4 - 0.25 = 1.5 |
C.
| Input | Output |
| 1 | 1 ÷ 4 - 0.25 = 4.75 |
| 2 | 2 ÷ 4 - 0.25 = 5.75 |
| 3 | 3 ÷ 4 - 0.25 = 6.75 |
| 4 | 4 ÷ 4 - 0.25 = 7.75 |
D.
| Input | Output |
| 1 | 1 ÷ 4 - 0.25 = 3.75 |
| 2 | 2 ÷ 4 - 0.25 = 7.75 |
| 3 | 3 ÷ 4 - 0.25 = 11.75 |
| 4 | 4 ÷ 4 - 0.25 = 15.75 |
Answer: A
You might also like to view...
Use Taylor's formula to find the requested approximation of 
near the origin.Quadratic approximation to 
A. 1 + 6x + y +36x2 + 6xy + y2 B. 1 - 6x - y + 36x2 + 6xy + y2 C. 1 + 6x + y + 36x2 + 12xy + y2 D. 1 - 6x - y + 36x2 + 12xy + y2
Find the solution set for each inequality. Graph the solution set on a number line and write it in interval notation.|x| + 4 ? 14
A. (-?, -10] ? [10, ?)
B. [10, ?)
C. [-10, 10]
D. [18, ?)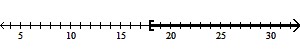
s(t) is the position of a body moving along a coordinate line, where t?0, and s(t) is measured in feet and t in seconds.
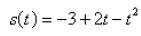
a. Determine the time(s) and the position(s) when the body is stationary.
b. When is the body moving in the positive direction? In the negative direction?
c. Sketch a schematic showing the position of the body at any time t.
Find the unknown value.48% of what number is 84?
A. 0.57 B. 57 C. 175 D. 1750