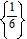Solve the problem.An express train and a local train leave a station at the same time (on separate tracks) and head for a town 50 miles away. The express travels twice as fast as the local and arrives 1 hour ahead of the local. Find the speed of each train.
A. Express: 100 mph; local: 50 mph
B. Express: 25 mph; local: 12.5 mph
C. Express: 50 mph; local: 25 mph
D. Express: 60 mph; local: 30 mph
Answer: C
You might also like to view...
Refer to the figures and recursive rules below to answer the following question(s).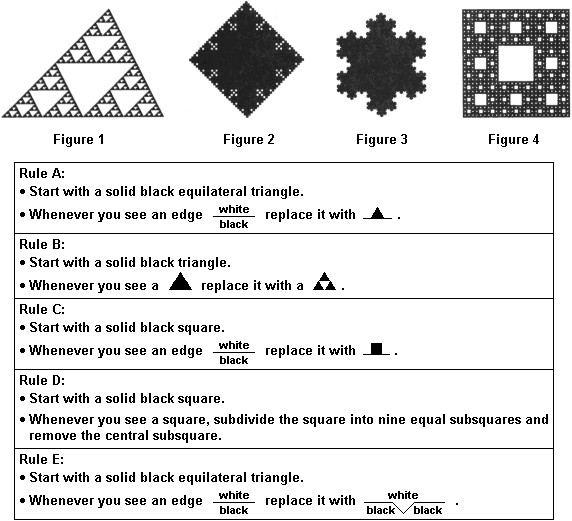
Which of the figures above approximates the result of recursively applying Rule A infinitely many times?
A. Figure 4 B. Figure 2 C. Figure 3 D. Figure 1 E. none of these
Find the sum or difference.-(2x3 + x - 8) + (4x3 + 2x2) - (9x2 - 3x -1)
A. 2x3 + 7x2 + 4x - 9 B. 2x3 - 7x2 + 2x + 9 C. 1x3 - 5x2 - 2x + 9 D. 6x3 - 11x2 + 2x - 9
Find an equation of the tangent line to the graph of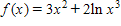 
at its inflection point.
What will be an ideal response?
Use the method of your choice to find all real solutions of the equation.x = -3 + 
A. {-3, -6}
B. No real solutions
C. {-6, 3}
D. 