Solve.A plane flying with a tailwind flew at a speed of 420 mph, relative to the ground. When flying against the tailwind, it flew at a speed of 300 mph. Express these relationships as equations. Let x represent the speed of the plane in calm air and let y represent the speed of the wind.
A. x + y = 420
y = 300
B. x + y = 300
x - y = 420
C. x + y = 300
y = 420
D. x + y = 420
x - y = 300
Answer: D
Mathematics
You might also like to view...
Convert the rectangular equation to polar form.x2 + y2= 144
A. r = 12 cos ? B. r = 12 C. r = 12 sin ? D. r = 144
Mathematics
Given functions f and g, determine the domain of f + g.f(x) = 3x - 7,g(x) = 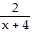
A. (-?, -2) or (-2, ?) B. (-?, -4) or (-4, ?) C. (-?, ?) D. (0, ?)
Mathematics
Simplify the expression. Assume that all variables are positive when they appear.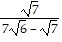
A. 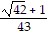
B. 
C. 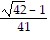
D. 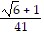
Mathematics
Find an equation of the parabola described.Focus at (4, 0); vertex at (0, 0)
A. x2 = 16y B. y2 = 4x C. x2 = 4y D. y2 = 16x
Mathematics