Replace the polar equation with an equivalent Cartesian equation.r2 + 2r2 sin ? cos ? = 324
A. x2 + y2 = 324
B. x + y = 18
C. x + y = ±18
D. x2 + 3y2 = 324
Answer: C
Mathematics
You might also like to view...
Use the properties of logarithms to find the exact value of the expression. Do not use a calculator.log98 14 + log98 7
A. 98 B. 1 C. 14 D. 7
Mathematics
Determine the degree and the coefficient of the monomial.12y7
A. Degree 7, coefficient 12 B. Degree 0, coefficient 0 C. Degree 8, coefficient - 7 D. Degree 12, coefficient 8
Mathematics
Approximate the area under the curve and above the x-axis using n rectangles. Let the height of each rectangle be given by the value of the function at the right side of the rectangle.f(x) = 2x2 + x + 3 from x = 0 to x = 6; n = 6
A. 211 B. 200 C. 230 D. 221
Mathematics
Find the area bounded by the curves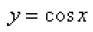 
and y=
between x=0 and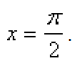 
What will be an ideal response?
Mathematics