Find the vertex, focus, and directrix of the parabola with the given equation.(y - 2)2 = 4(x + 4)
A. vertex: (4, -2)
focus: (5, -2)
directrix: x = 3
B. vertex: (-4, 2)
focus: (-5, 2)
directrix: x = -3
C. vertex: (-4, 2)
focus: (-3, 2)
directrix: x = -5
D. vertex: (2, -4)
focus: (3, -4)
directrix: x = 1
Answer: C
You might also like to view...
Use a finite sum to estimate the average value of the function on the given interval by partitioning the interval and evaluating the function at the midpoints of the subintervals.f(t) = 3 -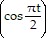 
2 on [0, 4] divided into 4 subintervals
A. 
B. 
C. 3
D.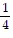 
Use the Binomial Theorem to find the indicated coefficient or term.The 4th term in the expansion of (x + 3y)10
A. 3240x7y3 B. 3240x3y7 C. 1080x7y4 D. 1080x3y7
Determine whether the ordered pair is a solution for the given equation.14x - 6y = -72; (-3, 5)
A. Yes B. No
Find the x- and y-intercepts of the graph.
A. (4, 0), (-4, 0), (0, 0) B. (0, -4) C. (4, 0), (-4, 0), (0, -4) D. (4, 0), (-4, 0)