Solve the problem.An initial deposit of $45,000 is made into an account that earns 5.4% compounded continuously. Money is then withdrawn at a constant rate of $2,700 a year until the amount in the account is 0. Find the amount in the account at any time t. When is the amount 0? What is the total withdrawn from the account?
A. The amount y in the account at any time t is y = 50,000 - 5,000e0.054t. The account will be depleted after about 20.5 years. A total of about $55,350 will have been withdrawn at that time.
B. The amount y in the account at any time t is y = 50,000 - 5,000e0.054t. The account will be depleted after about 42.6 years. A total of about $115,130 will have been withdrawn at that time.
C. The amount y in the account at any time t is y = 50,000 - 7,500e0.054t. The account will be depleted after about 15.4 years. A total of about $41,580 will have been withdrawn at that time.
D. The amount y in the account at any time t is y = 50,000 - 7,500e0.054t. The account will be depleted after about 35.1 years. A total of about $94,770 will have been withdrawn at that time.
Answer: B
You might also like to view...
Evaluate.Find (f + g)(-1) when f(x) = x + 5 and g(x) = x + 4.
A. -11 B. 7 C. -1 D. -3
Sketch the curve.4x2 + 25y2 = 100
A. 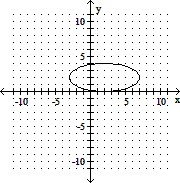
B. 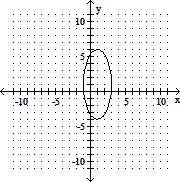
C. 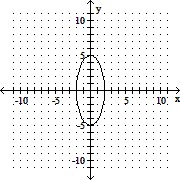
D. 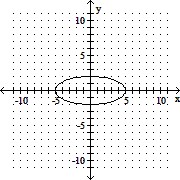
Simplify. Your answer should be in lowest terms.- 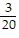 +
+ 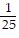
A. - 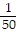
B. - 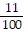
C. 
D. 
Solve the problem.If f(x) = 2x, find f[log2 (ln 2)].
A. 0 B. 2ln 2 C. log2 2 D. ln 2