Solve the linear programming problem.The Fiedler family has up to $130,000 to invest. They decide that they want to have at least $40,000 invested in stable bonds yielding 5.5% and that no more than $60,000 should be invested in more volatile bonds yielding 11%. How much should they invest in each type of bond to maximize income if the amount in the stable bond should not exceed the amount in the more volatile bond? What is the maximum income?
What will be an ideal response?
$60,000 in the stable bonds and $60,000 in the volatile bonds; maximum income $9900
You might also like to view...
Find average for each set of numbers.5, 15, 11, 13, 15, 14, 5, 18
A. 11 B. 13.71 C. 23 D. 12
Add or subtract.5.9 + 8 + 9.7
A. 22.6 B. 23.4 C. 23.6 D. 22.4
Use the percentage method of withholding, a FICA rate of 6.2%, a Medicare rate of 1.45%, an SDI rate of 1%, and a state withholding tax of 3.4%. One withholding allowance is $73.08 when paid weekly.
Larry Calanan has earnings of $510 in a week. He is single and claims 2 withholding allowances. His deductions include FICA, Medicare, federal withholding, state disability insurance, state withholding, union dues of $15, and charitable contributions of $21. Find his net pay.
A. $361.54 B. $389.85 C. $408.51 D. $372.51
Find the mean, median, mode, and midrange for the given data. Round to the nearest tenth if necessary.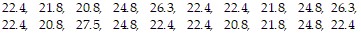
A. mean = 23.2, median = 22.4, mode = 22.4, midrange = 24.15 B. mean = 23.2, median = 22.4, mode = 22.4, midrange = 6.7 C. mean = 23.2, median = 24.8, mode = 22.4, midrange = 24.15 D. mean = 23.7, median = 22.4, mode = 27.5, midrange = 6.7