Solve the equation.(x + 5)(x - 2)(x - 15) = 0
A. {5, 2, 15}
B. {0, -5, 2}
C. {-5, 2, 15}
D. {-5, 2, -15}
Answer: C
You might also like to view...
Use the addition property of equality to solve the equation.-15 = 1 + a
A. 16 B. -14 C. 14 D. -16
Solve the problem.Matthew has two different stocks. One of the stocks is worth $7 more per share than the other. He has 13 shares of the more valuable stock and 31 shares of the other stock. His total assets in stocks is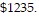 
How much is the more expensive stock worth per share?
A. $33 per share B. $19 per share C. $35 per share D. $7 per share
Use the properties of limits to help decide whether the limit exists. If the limit exists, find its value.
A. 
B. ?
C. - 
D. 0
In a laboratory experiment, two separate foods are given to experimental animals. Each food contains essential ingredients, A and B, for which the animals have a minimum requirement, and each food also has an ingredient C, which can be harmful to the animals. The table below summarizes this information. ? Food 1 Food 2 Required Ingredient A 10 units/g 3 units/g 33 units Ingredient B 6 units/g 15 units/g 33 units Ingredient C 5 units/g 1 unit/g ? Determine how many grams of foods 1 and 2 should be given in order to satisfy the requirements for A and B while minimizing the amount of ingredient C ingested. Also determine the minimum amount of ingredient C ingested. Round your answer to one decimal place if necessary. ?
A. 11 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams. B. ?0 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 11 grams. C. ?12 grams of food 1 and 0 gram0 of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 60 grams. D. ?1 gram0 of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 16 grams. E. ?2 grams of food 1 and 11 grams of food 2 satisfy the minimum requirements for A and B while minimizing the amount of ingredient C to 21 grams.