State the dual of the linear programming problem.Maximize3x1?+ 8x2+ 3x3 subject to3x1?+x3?? 224x1?+ 3x2+ x3? 43and x1 ? 0, x2 ? 0, x3 ? 0
A.
| Minimize | 22y1? | + 43y2 |
| subject to | 3y1 | + 4y2 | ? 3 |
| ? | ? | 3y2? 8 |
| y1? | + y2 | ? 3 |
B.
| Minimize | 3y1? | + 8y2 | + 3y3 |
| subject to | 3y1? | + | y3? | ? 22 |
| 4y1? | + 3y2 | + y3 | ? 43 |
C.
| Minimize | 22y1? | + 43y2 |
| subject to | 3y1 | + 4y2 | ? 3 |
| ? | ? | 3y2? 8 |
| y1? | + y2 | ? 3 |
D.
| Minimize | 3y1? | + 8y2 |
| subject to | 3y1 | + 4y2 | ? 22 |
| ? | ? | 3y2? 43 |
| y1? | + y2 | ? 3 |
Answer: C
Mathematics
You might also like to view...
Graph the function f(x) over the given interval. Partition the interval into 4 subintervals of equal length. Then add to your sketch the rectangles associated with the Riemann sum 
, using the indicated point in the kth subinterval for ck.f(x) = x2 - 3, [0, 8], midpoint
A. 
B. 
C.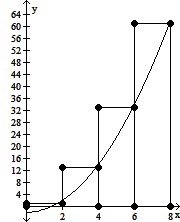 
D.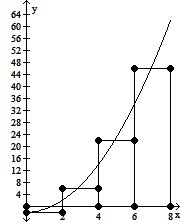 
Mathematics
Provide an appropriate response.If x3 ? f(x) ? x for x in [-1,1], find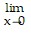 
f(x) if it exists.
A. 0 B. -1 C. does not exist D. 1
Mathematics
Give the amplitude or period as requested.Amplitude of y = -4 sin x
A. 4
B. 2?
C. -4?
D. 
Mathematics
Provide the proper response. Show all fractions in simplest form.Refer to the figure below and represent the indicated amount as a fraction.
The dots inside the circle as a part of all the dots.
A. 
B.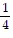 
C. 
D.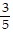 
Mathematics