Solve the problem.A painting was sold in 1985 for $1 million. The painting was then resold in 1996 for $6 million. Assume that the painting's value increases exponentially. Find the exponential growth rate k, and determine the exponential growth function, assuming V0 = 1. (Round decimals to three places.)
A. k = 0.163; V(t) = 1e0.163, where V(t) is in millions and t is the number of years after 1985.
B. k = 0.163; V(t) = 1e0.163t, where V(t) is in millions and t is the number of years after 1985.
C. k = 0.163; V(t) = 1e0.163t, where V(t) is in millions and t is the number of years after 1997.
D. k = 0.149; V(t) = 1e0.149t, where V(t) is in millions and t is the number of years after 1985.
Answer: B
You might also like to view...
Find the product.
? 
A. 
B. 
C. 
D. 
Find y in terms of x.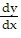
= 5x-3/4, curve passes through (1, 8)
A. y = 5x1/4 + 3
B. y = 20x1/4 + 160
C. y =-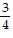 
x-7/4 - 3
D. y = 20x1/4 - 12
Add. Simplify, if possible.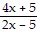
+ 
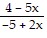
A. 
B. - 
x -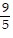 
C. 
D.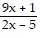 
Solve the problem.The perimeter of a rectangle is 70 m. If the width were doubled and the length were increased by 24 m, the perimeter would be 138 m. What are the length and width of the original rectangle?
A. Width: 10 m; length: 25 m B. Width: 17 m; length: 17 m C. Width: 25 m; length: 10 m D. Width: 12 m; length: 17 m