Test the validity of the argument. Tell and show why the argument is valid or invalid.If it is warm, Brenda will go to the park or go shopping. It is warm and Brenda goes shopping. Thus, she does not go to the park.
What will be an ideal response?
| Let | w = "It is warm" |
s = "Brenda will go shopping"
If the argument is valid, the implication is a tautology. The only time that the implication could be false is if the hypothesis is true and the conclusion is false. Consider the case where p, w, and s are true, and fill in the truth values in evaluating the statement.
[(w ? (p ? s)) ? (w ? s)] ? ~p
| T T | T | T | T | F F |
| (1) (3) (2) | (5) | (4) | (7) (6) |
Mathematics
You might also like to view...
Solve the problem.If 11 newborn babies are randomly selected, how many different gender sequences are possible?
A. 121 B. 22 C. 39,916,800 D. 2048
Mathematics
Find the linear function that corresponds to the graph or passes through the given points. 
A. y = -6x - 2
B. y = 6x - 2
C. y = 3x + 6
D. y =  x - 2
x - 2
Mathematics
Graph the inequality.x + 4y < 6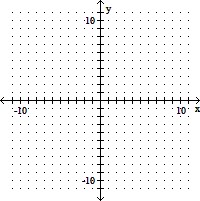
A. 
B. 
C. 
D. 
Mathematics
Solve.A = 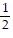 bh for h
bh for h
A. h = 
B. h = 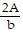
C. h = 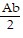
D. h = 
Mathematics