Solve the problem.Given an algae population in a certain pond, x (in millions), f(x) models the frog population in the pond (in thousands). (i) Use the graph of f to estimate the number of algae in the pond when there are 29 thousand frogs in the pond; (ii) Estimate f(x) at 2, 3, 5, 6 and 7; (iii) Use regression and the points you estimated to find a quartic polynomial function f that models the data points; (iv) Use f(x) to solve part (i) either graphically or numerically. 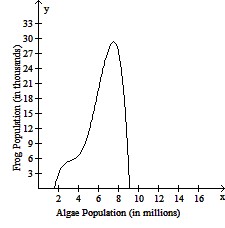
A. (i) about 1 million
(ii) 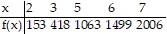 (approximately; answers may vary);
(approximately; answers may vary);
(iii) f(x) ? -0.18x4 + 3.27x3 + 20.02x2 + 55.93x - 46.00;
(iv) x ? 1.1 million algae when f(x) = 29 thousand frogs
B. (i) about 1 million
(ii) 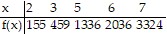
(iii) f(x) ? 0.16x4 + 3.77x3 + 20.02x2 + 51.93x - 44.00;
(iv) x ? 1.5 million algae when f(x) = 29 thousand frogs
C. (i) about 5 million
(ii) 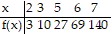 (approximately; answers may vary);
(approximately; answers may vary);
(iii) f(x) ? -0.18x4 + 3.77x3 - 20.02x2 + 41.93x - 44.00;
(iv) x ? 5.1 million algae when f(x) = 29 thousand frogs
D. (i) about 7 million
(ii)  (approximately; answers may vary);
(approximately; answers may vary);
(iii) f(x) ? -0.18x4 + 3.27x3 - 20.02x2 + 51.93x - 44.00;
(iv) x ? 7.3 million algae when f(x) = 29 thousand frogs
Answer: D
You might also like to view...
The equation gives the position s = f(t) of a body moving on a coordinate line (s in meters, t in seconds). s (m)
 When is the body standing still?
When is the body standing still?
A. 8 < t ? 10 B. 2 < t < 3, 4 < t < 5, 7 < t < 9 C. t = 3, t = 5, t = 8 D. 1 < t < 2
Use a table to answer the question. Round to the nearest percent.A population is normally distributed. What percentage of the population lies to the left of the population z score 0.66?
A. 77% B. 69% C. 72% D. 75%
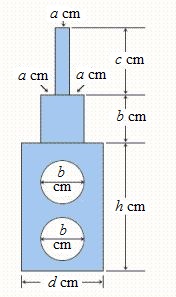
Find the distance around the figure below by using the appropriate formula.
?
Fill in the blank(s) with the appropriate word(s).
Subtract.(2a + 2b - c) - (8b + 4c + 6d)
A. 2a - 6b - 3c - 6d B. 2a - 6b + 5c + 6d C. 2a - 6b - 5c - 6d D. 2a - 6b - 5c + 6d