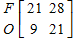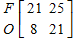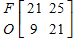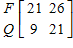Determine whether the given sequence is arithmetic, geometric, or neither. If the sequence is arithmetic, find the common difference; if it is geometric, find the common ratio.{5n - 3}
A. Arithmetic; d = -3
B. Arithmetic; d = 5
C. Geometric; r = 5
D. Neither
Answer: B
You might also like to view...
Solve the system. If there is no solution or if the system's equations are dependent, so state.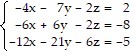
A. no solution or ? B. {(3, 3, -1)} C. {(2, -8, -5)} D. infinitely many solutions; dependent equations
Find the median of the list of data. ? 15, 18, 20, 29, 34, 44 ?
A. 22.5 B. 29 C. 20 D. 24.5 E. 26.7
Simplify and write the answer using scientific notation.(6.22 × 102)(6.50 × 102)
A. 4.04 × 105 B. 4.04 × 104 C. 6.50 × 104 D. 6.50 × 105
Florida and Ohio are "swing states" that have a large bounty of electoral votes and are therefore highly valued by presidential campaign strategists. Suppose it is now the weekend before Election Day 2004, and each candidate (Bush and Kerry) can visit only one more state. Further, to win the election, Bush needs to win both of these states. Currently Bush has a 70% chance of winning Ohio and a 30% chance of winning Florida. Therefore, he has a 0.70×0.30 = 0.21, or 21% chance of winning the election. Assume that each candidate can increase his probability of winning a state by 20% if he, and not his opponent, visits that state. If both candidates visit the same state, there is no effect. Set up a payoff matrix with Bush as the row player and Kerry as the column player, where the payoff
for a specific set of circumstances is the probability (expressed as a percentage) that Bush will win both states.
?
A. Kerry?
F O
Bush
B. Kerry
F O
?Bush
C. Kerry
F O
?Bush
D. Kerry
F O?
?Bush
E. Kerry
? F O
?Bush